녹원학원 수학과학영재교육 010-3549-5206

(풀이)
(풀이 1)삼각형 CBD 의 넓이는 3*3/2 =9/2
삼각형 DKE 의 넓이는 9/8
삼각형 ELF 의 넓이는 9/32

색칠한 삼각형의 합은 9/2 + 9/8 + 9/32 = 189/32 = 5.9

삼각형 FGH 를 모두 색칠하면 9/32 만큼 더 더하면 된다.
합은 9/2 + 9/8 + 9/32 + 9/32 = 198/32 = 6.2

색칠한 삼각형의 전체 넓이는 5.9 와 6.2 사이에 있다.
답은 ( A) 6 이다.

(풀이 2)
사다리꼴 ACDJ 는 삼각형 3개중 한개인 BCD 에 색칠되어 있고,
사다리꼴 JDEI 는 삼각형 3개중 한개인 KDE 에 색칠되어 있고,
사다리꼴 IEFH 는 삼각형 3개중 한개인 LEF 에 색칠되어 있다.

이렇게 100번을 반복하면 각 사다리꼴의 1/3 이 색칠되어 있고 모두 합하면
삼각형 ACG의 넓이 6*6/2 = 18 의 3분의 1인 18/3 = 6 이다.

(풀이 3)
색칠한 삼각형의 전체 넓이는
9/2 + 9/8 + 9/32 + 9/128 + ............. = ?

a1 = 9/2, r = 1/4
초항 9/2 이고 , 공비 1/4 이다.

무한급수의 합은 S = a1/( 1- r ) = (9/2) / ( 1 - 1/4 ) = 6

(풀이 4)
변 AC 에 한점을 잡고 점 P라 하자.
점 P 와 점 G를 연결하면 대략 삼각형을 3등분 함을 알수있다.
((6*6)/2)/3 = 6

(풀이 5)

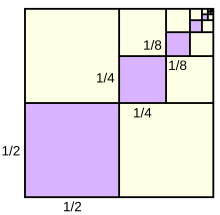
Each of the purple squares has 1/4 of the area of the next larger square
(1/2×1/2 = 1/4, 1/4×1/4 = 1/16, etc.).
The sum of the areas of the purple squares
is one third of the area of the large square.

Points B, D, and J are midpoints of the sides of right triangle ACG. Points K, E, I are midpoints of the sides of triangle JDG, etc. If the dividing and shading process is done 100 times (the first three are shown) and AC = CG = 6, then the total area of the shaded triangles is nearest

(A) 6 (B) 7 (C) 8 (D) 9 (E) 10

Solution

Solution 1

Since FGH is fairly small relative to the rest of the diagram, we can make an underestimate by using the current diagram. All triangles are right-isosceles triangles.

$CD = \frac {CG}{2} = 3, DE = \frac{CD}{2} = \frac{3}{2}, EF = \frac{DE}{2} = \frac{3}{4}$

$CB = CD = 3, DK = DE = \frac{3}{2}, EL = EF = \frac{3}{4}$

$[CBD] = \frac{1}{2}3^2 = \frac{9}{2}$

$[DKE] = \frac{1}{2}(\frac{3}{2})^2 = \frac{9}{8}$

$[ELF] = \frac{1}{2}(\frac{3}{4})^2 = \frac{9}{32}$

The sum of the shaded regions is $\frac{9}{2} + \frac{9}{8} + \frac{9}{32} = \frac{189}{32} \approx 5.9$

$5.9$ is an underestimate, as some portion (but not all) of $\triangle FGH$ will be shaded in future iterations.

If you shade all of $\triangle FGH$ , this will add an additional $\frac{9}{32}$ to the area, giving $\frac{198}{32} \approx 6.2$ , which is an overestimate.

Thus, $6 \rightarrow \boxed{A}$ is the only answer that is both over the underestimate and under the overestimate.

Solution 2

In iteration $1$ , congruent triangles $\triangle ABJ, \triangle BDJ,$ and $\triangle BDC$ are created, with one of them being shaded.

In iteration $2$ , three more congruent triangles are created, with one of them being shaded.

As the process continues indefnitely, in each row, $\frac{1}{3}$ of each triplet of new congruent triangles will be shaded. The "fourth triangle" at the top ( $\triangle FGH$ in the diagram) will gradually shrink,

leaving about $\frac{1}{3}$ of the area shaded. This means $\frac{1}{3}\left(\frac{1}{2}6\cdot 6\right) = 6$ square units will be shaded when the process goes on indefinitely, giving $\boxed{A}$ .

Solution 3

Using Solution 1 as a template, note that the sum of the areas forms a geometric series:

$\frac{9}{2} + \frac{9}{8} + \frac{9}{32} + \frac{9}{128} + ...$

This is the sum of a geometric series with first term $a_1 = \frac{9}{2}$ and common ratio $r = \frac{1}{4}$ This is the easiest way to do this problem.

The sum of an infinite geometric series with $|r|<1$ is shown by the formula. $S_{\infty} = \frac{a_1}{1 - r}$ Insert the values to get $\frac{\frac{9}{2}}{1 - \frac{1}{4}} = \frac{9}{2}\cdot\frac{4}{3} = 6$ , giving an answer of $\boxed{A}$ .

Art of Problem Solving

궁금한게 있으면 010-3549-5206으로..

녹원학원 수학과학영재교육 010-3549-5206

2019년 9월 6일 금요일

수학의재미 1999 AMC 8 문제 25 등비수열

Solution

Solution 1

Solution 2

Solution 3

댓글 없음: