아폴로니오스의 정리-중선정리
초등 기하학에서 아폴로니오스의 정리(Apollonius' theorem)는 삼각형의 각 변들간의 관계를 설명한 정리이다. 중선정리라는 이름으로도 널리 알려져 있고, 흔히 파푸스의 중선정리라는 이름으로 알려져 있으나 이 정리에 파푸스(Pappus)의 이름을 붙여 부르는 예는 대한민국과 일본 이외에는 찾을 수 없다. '아폴로니오스'라는 이름은 고대 그리스의 수학자인 페르게의 아폴로니오스의 이름을 딴 것이다.
그림에서 
일 때, 선분 
는 중선(Median)이 되고, 다음의 관계가 성립한다.
가 성립할 경우, 피타고라스의 정리가 된다. 즉,
를 가정할 때와 동일하므로 스튜어트 정리의 특수한 형태가 된다.
정리의 서술
일 때, 선분 는 중선(Median)이 되고, 다음의 관계가 성립한다.
가 성립할 경우, 피타고라스의 정리가 된다. 즉,
를 가정할 때와 동일하므로 스튜어트 정리의 특수한 형태가 된다.
Apollonius' theorem
Area of Green + Area of Purple = Area of Red
In geometry, Apollonius' theorem is a theorem relating the length of a median of a triangle to the lengths of its side. Specifically, in any triangle ABC, if AD is a median, then
It is a special case of Stewart's theorem. For an isosceles triangle the theorem reduces to the Pythagorean theorem. From the fact that diagonals of a parallelogram bisect each other, the theorem is equivalent to the parallelogram law.
The theorem is named for Apollonius of Perga.
Proof
Proof of Apollonius' theorem
The theorem can be proved as a special case of Stewart's theorem, or can be proved using vectors (see parallelogram law). The following is an independent proof using the law of cosines.[1]
Let the triangle have sides a, b, c with a median d drawn to side a. Let m be the length of the segments of a formed by the median, so m is half of a. Let the angles formed between a and d be θ and θ′ where θ includes b and θ′ includes c. Then θ′ is the supplement of θ and cos θ′ = −cos θ. The law of cosines for θ and θ′ states
Add these equations to obtain
as required.
Wikipedia
If O be the mid-point of the side MN of the triangle LMN, then
LM2 + LN2 = 2(LO2 + MO2).
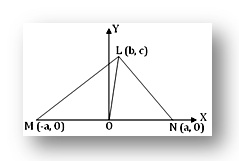
Proof: Let us choose origin of rectangular Cartesian co-ordinates at O and x-axis along the side MN and OY as the y – axis . If MN = 2a then the co-ordinates of M and N are (- a, 0) and (a, 0) respectively. Referred to the chosen axes if the co-ordinates of L be (b, c) then
LO2 = (b - 0)2 + (C - 0)2 , [Since, co- ordinates of O are (0, 0)]
= b2 + c2;
MO2 = (- a - 0)2 + (0 – 0)2 = a2LMB2 = (b + a) 2 + (c – 0)2 = (a + b)2 + c2And LN2 = (b - a) 2 + (c - 0) 2 = (a - b)2 + c2Therefore, LM2 + LN2 = (a + b) 2 + c2 + (b - a)2 + c2
= 2(a2 + b2) + 2c2 = 2a2 + 2(b2 + c2)
= 2MO2 + 2LO2
= 2(MO2 + LO2).
= 2(LO2 + MO2).
Apollonius' theorem
The theorem is named for Apollonius of Perga.
Proof
Let the triangle have sides a, b, c with a median d drawn to side a. Let m be the length of the segments of a formed by the median, so m is half of a. Let the angles formed between a and d be θ and θ′ where θ includes b and θ′ includes c. Then θ′ is the supplement of θ and cos θ′ = −cos θ. The law of cosines for θ and θ′ states
Wikipedia
If O be the mid-point of the side MN of the triangle LMN, then
LM2 + LN2 = 2(LO2 + MO2).
Proof: Let us choose origin of rectangular Cartesian co-ordinates at O and x-axis along the side MN and OY as the y – axis . If MN = 2a then the co-ordinates of M and N are (- a, 0) and (a, 0) respectively. Referred to the chosen axes if the co-ordinates of L be (b, c) then
LO2 = (b - 0)2 + (C - 0)2 , [Since, co- ordinates of O are (0, 0)]
= b2 + c2;
MO2 = (- a - 0)2 + (0 – 0)2 = a2LMB2 = (b + a) 2 + (c – 0)2 = (a + b)2 + c2And LN2 = (b - a) 2 + (c - 0) 2 = (a - b)2 + c2Therefore, LM2 + LN2 = (a + b) 2 + c2 + (b - a)2 + c2
= 2(a2 + b2) + 2c2 = 2a2 + 2(b2 + c2)
= 2MO2 + 2LO2
= 2(MO2 + LO2).
= 2(LO2 + MO2).
If O be the mid-point of the side MN of the triangle LMN, then
LM2 + LN2 = 2(LO2 + MO2).
Proof: Let us choose origin of rectangular Cartesian co-ordinates at O and x-axis along the side MN and OY as the y – axis . If MN = 2a then the co-ordinates of M and N are (- a, 0) and (a, 0) respectively. Referred to the chosen axes if the co-ordinates of L be (b, c) then
LO2 = (b - 0)2 + (C - 0)2 , [Since, co- ordinates of O are (0, 0)]
= b2 + c2;
MO2 = (- a - 0)2 + (0 – 0)2 = a2LMB2 = (b + a) 2 + (c – 0)2 = (a + b)2 + c2And LN2 = (b - a) 2 + (c - 0) 2 = (a - b)2 + c2Therefore, LM2 + LN2 = (a + b) 2 + c2 + (b - a)2 + c2
= 2(a2 + b2) + 2c2 = 2a2 + 2(b2 + c2)
= 2MO2 + 2LO2
= 2(MO2 + LO2).
= 2(LO2 + MO2).
댓글 없음:
댓글 쓰기