Incenter

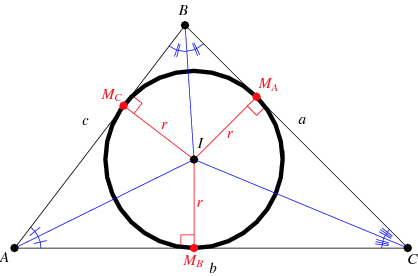
The incenter 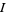 is the center of the incircle for a polygon or insphere for a polyhedron (when they exist). The corresponding radius of the incircle or insphere is known as the inradius.

The incenter can be constructed as the intersection of angle bisectors. It is also the interior point for which distances to the sides of the triangle are equal. It has trilinear coordinates 1:1:1, i.e., triangle center function

(1)

and homogeneous barycentric coordinates . It is Kimberling center .

For a triangle with Cartesian vertices , , , the Cartesian coordinates of the incenter are given by

(2)

The distance between the incenter and circumcenter is 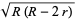, where  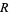is the circumradius and  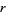is the inradius, a result known as the Euler triangle formula.

The incenter lies on the Nagel line and Soddy line, and lies on the Euler line only for an isosceles triangle. The incenter is the center of the Adams' circle, Conway circle, and incircle. It lies on the Darboux cubic,M'Cay cubic, Neuberg cubic, orthocubic, and Thomson cubic. It also lies on the Feuerbach hyperbola.

For an equilateral triangle, the circumcenter 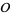, triangle centroid 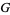, nine-point center 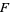, orthocenter 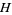, and de Longchamps point  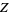all coincide with .

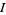The distances between the incenter and various named centers are given by

			(3)
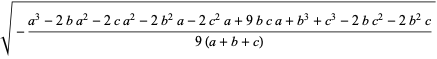			(4)
			(5)
			(6)
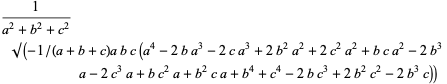			(7)
			(8)
			(9)
			(10)
			(11)
			(12)
			(13)
			(14)

where 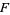 is the Feuerbach point,  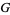is the triangle centroid,  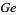is the Gergonne point,  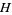is the orthocenter,  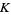is the symmedian point,  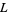is the de Longchamps point,  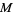is the mittenpunkt,  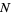is the nine-point center,  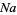is the Nagel point,  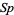is the Spieker center,  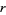is the inradius,  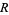is the circumradius,  is the triangle area, and 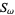 is Conway triangle notation.

The following table summarizes the incenters for named triangles that are Kimberling centers.

triangle	Kimberling	incenter
anticomplementary triangle		Nagel point
circumnormal triangle		circumcenter
circum-orthic triangle		orthocenter
circumtangential triangle		circumcenter
contact triangle		first mid-arc point
Euler triangle		midpoint of  and
excentral triangle		incenter of excentral triangle
extangents triangle		Bevan point
first Morley triangle		first Morley center
first Yff circles triangle		incenter
inner Napoleon triangle		triangle centroid
intangents triangle		incenter
Lucas central triangle		isogonal conjugate of
medial triangle		Spieker center
orthic triangle		orthocenter
outer Napoleon triangle		triangle centroid
reference triangle		incenter
second Yff circles triangle		incenter
Stammler triangle		circumcenter
tangential mid-arc triangle		incenter
tangential triangle		circumcenter

The incenter and excenters of a triangle are an orthocentric system.

The circle power of the incenter with respect to the circumcircle is

(15)

(Johnson 1929, p. 190).

If the incenters of the triangles , , and  are , , and , then  is equal and parallel to , where  are the feet of the altitudes and  are the incenters of thetriangles. Furthermore, , , , are the reflections of  with respect to the sides of the triangle  (Johnson 1929, p. 193).

SEE ALSO:Circumcenter, Cyclic Quadrilateral, Excenter, Gergonne Point, Incentral Triangle, Incircle, Inradius, Orthocenter, Orthocentric Centroid, Nagel Line

Wolfram