녹원학원 수학과학영재교육 010-3549-5206 : 삼각형의 합동(合同, congruence)

2019년 2월 6일 수요일

삼각형의 합동(合同, congruence)

정의

등거리 변환은 두 점 사이의 거리를 보존하는 변환이다.

\mathbb {R} ^{n}

의 두 도형

M,N\subseteq \mathbb {R} ^{n}

이 다음 조건을 만족시키면,

M,N

이 합동이라고 한다.

$N=I(M)$ 인 등거리 변환 $I\colon \mathbb {R} ^{n}\to \mathbb {R} ^{n}$ 이 존재한다.

도형의 합동은 동치 관계를 이룬다. 도형의 합동은 닮음에서 닮음비가 1인 특수한 경우다.

기하학에서, 합동(合同, 영어: congruence)은 두 도형이 모양과 크기가 같음을 나타내는 관계이다. 즉, 두 도형을 점집합으로 생각할 때, 하나에 어떤 등거리 변환에 대한 상을 취하여 다른 하나를 얻을 수 있다면, 두 도형이 합동이라고 한다. 서로 합동인 도형은 서로 닮음이다. 그러나 그 역은 일반적으로 성립하지 않는다.

성질

삼각형의 합동

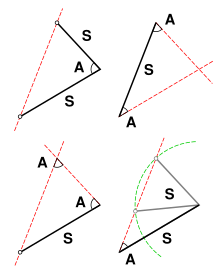
평면 삼각형은 합동 조건 SAS, ASA, AAS를 갖지만, 합동 조건 SSA를 갖지 않는다.

두 삼각형이 합동이라면, 이 두 삼각형의 세 쌍의 변(의 길이) 및 세 쌍의 각(의 크기)은 각각 같다. 각 쌍의 변을 대응변(對應邊, 영어: corresponding sides)이라고 하며, 각 쌍의 각을 대응각(對應角, 영어: corresponding angles)이라고 한다.

삼각형

ABC

와 삼각형

DEF

의 합동은 기호로 다음과 같이 나타낸다.

\triangle ABC\cong \triangle DEF

단, 같은 위치의

A

와

D

B

와

E

C

와

F

는 대응점이어야 한다.^[1]^:5

두 삼각형

ABC,DEF

가 합동일 몇 가지 충분 조건은 다음과 같다.

SSS(변변변): 만약 $AB=DE$ , $AC=DF$ , $BC=EF$ 라면, $\triangle ABC\cong \triangle DEF$ 이다. 즉, 두 삼각형의 세 쌍의 대응변이 각각 같다면, 두 삼각형은 합동이다.
SAS(변각변): 만약 $AB=DE$ , $AC=DF$ , $\angle A=\angle D$ 라면, $\triangle ABC\cong \triangle DEF$ 이다. 즉, 두 삼각형의 두 쌍의 대응변 및 그 사잇각이 각각 같다면, 두 삼각형은 합동이다.
ASA(각변각): 만약 $\angle A=\angle D$ , $\angle B=\angle E$ , $AB=DE$ 라면, $\triangle ABC\cong \triangle DEF$ 이다. 즉, 두 삼각형의 두 쌍의 대응각 및 그 공공변이 각각 같다면, 두 삼각형은 합동이다.
AAS(각각변): 만약 $\angle A=\angle D$ , $\angle B=\angle E$ , $BC=EF$ 라면, $\triangle ABC\cong \triangle DEF$ 이다. 즉, 두 삼각형의 두 쌍의 대응각 및 그 공공변이 아닌 변이 각각 같다면, 두 삼각형은 합동이다.
RHS: 만약 $\angle C=\angle D=90^{\circ }$ , $AB=DE$ , $AC=DF$ 라면, $\triangle ABC\cong \triangle DEF$ 이다. 즉, 두 직각 삼각형의 빗변과 한 직각변이 각각 같다면, 두 직각 삼각형은 합동이다.

그러나, 다음 조건 가운데 하나를 만족시키는 두 삼각형

ABC,DEF

는 합동일 필요가 없다.

SSA(변변각): 만약 $AB=DE$ , $BC=EF$ , $\angle C=\angle F$ 이더라도, $\triangle ABC\ncong \triangle DEF$ 일 수 있다. 즉, 두 쌍의 대응변 및 그 사잇각이 아닌 한 쌍의 각이 같더라도, 두 삼각형은 합동이 아닐 수 있다. 다만, 이 각이 직각일 경우, RHS에 따라 합동이다.
AAA(각각각): 만약 $\angle A=\angle D$ , $\angle B=\angle E$ , $\angle C=\angle F$ 이더라도, $\triangle ABC\ncong \triangle DEF$ 일 수 있다. 즉, 세 쌍의 대응각기 같더라도, 두 삼각형은 합동이 아닐 수 있다. 다만 이 경우 두 삼각형은 서로 닮음이다.
위키

댓글 없음:

댓글 쓰기