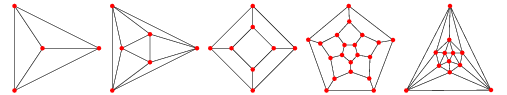
A polyhedral graph corresponding to the skeleton of a Platonic solid. The five platonic graphs, the tetrahedral graph, cubical graph, octahedral graph, dodecahedral graph, and icosahedral graph, are illustrated above. They are special cases of Schlegel graphs.
The following table summarizes the Platonic graphs and some of their properties.
| graph |
regularity | Hamiltonian | Eulerian | vertex-transitive | edge-transitive | |||
| cubical graph | cubic | 8 | 12 | 48 | yes | no | yes | yes |
| dodecahedral graph | cubic | 20 | 30 | 120 | yes | no | yes | yes |
| icosahedral graph | quintic | 12 | 30 | 120 | yes | no | yes | yes |
| octahedral graph | quartic | 6 | 12 | 48 | yes | yes | yes | yes |
| tetrahedral graph | cubic | 4 | 6 | 24 | yes | no | yes | yes Wolfram |
댓글 없음:
댓글 쓰기