“Do not worry about your difficulties in Mathematics. I can assure you mine are still greater.”
Mathematician: It is
impossible to know precisely what Einstein meant by this quote without being
able to ask him. It is often taken to mean that he did not have a strong
understanding of math or that he was bad at it when he was young. It is simply
wrong, however, to say that Einstein was bad at math. Some of his papers were
quite mathematically sophisticated, involving advanced subjects such as
stochastic differential equations and tensor calculus. What’s more, he excelled in math as a youth.
The following Einstein quote may help us gain a bit more perspective:
“Since the mathematicians have invaded the theory of relativity, I do not understand it myself anymore.”
(source: In A. Sommerfelt “To Albert Einstein’s Seventieth Birthday” in Paul A. Schilpp (ed.) Albert Einstein, Philosopher-Scientist, Evanston, 1949.)
Perhaps what Einstein meant when he claimed to have difficulties in math is that he felt as though it was a struggle to learn some of the very advanced math necessary for formulating his theories, or that (compared to mathematicians or mathematical physicists) his math skills were not exemplary. However, he certainly was far, far more gifted at math than your average person. All of this being said, the original quote was actually directed at young students, so it may have reflected little more than an attempt to encourage them to persevere despite their perceived difficulties.
Physicist: Here’s my guess.
Einstein witnessed the end of what might be considered the “intuitive physics”
of the 19th century and before. Most of that was his own damn fault. In 1905
(Einstein’s “miracle year“) he introduced the world to
both quantum mechanics (QM) and special relativity. Up until that time (most
of) modern physics was something that could be easily pictured and intuited.
You can draw the trajectories of moving objects, electric and magnetic fields
can be modeled using field lines, you can picture heat flowing like a fluid,
etc.
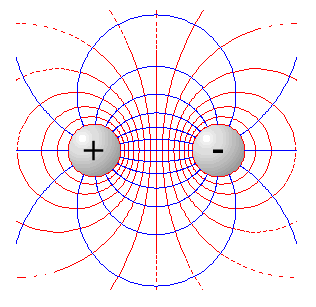
Electric field lines (in blue). Easy to
picture.
Moreover, the math involved is fairly simple. As in High School simple.
With the advent of relativity and QM came an age of science where the
conclusions being reached fell entirely out of the math. Nowadays, not only is
intuition pretty useless, but it will actively lead you wrong more often than
not.
Some of the first things we learned in relativity and QM are: there’s no
such thing as “now” for two different points, particles are actually waves,
those waves are actually particles, going fast makes time slow down, going fast
makes lengths shorter, energy and matter are the same stuff, space and time are
nearly the same, sometimes things will suddenly appear on the other side of
barriers they can’t get through, and on and on…
None of these things could be predicted intuitively, and many of them were
the result of some pretty tricky math. The brand of physics that’s practiced
today (the Standard Model, String Theory, and whatnot) involve math that’s so
crazy hard that it takes many years of training before you can even start to
grasp what’s going on. Einstein had many years of training, but could not have
imagined the tremendous variety of different maths that would come into play
because of his theories.
It was this last leap
in complexity that threw Einstein. Homeboy was really smart, really really good
at math, and a snappy dresser. However, even the people you would consider to
be unimaginably intelligent (I’m talking about the ‘Stein here) are almost
always over shadowed by someone way smarter, and with better, more specific,
preparation. Although he had a solid physics background, it was up to the
mathematicians (with their math and their glasses) to move the science forward.
Another
Physicist: I am not familiar with the quote. I only have a wild guess.
In the period between the publication of special relativity and general
relativity he took some time to learn enough differential geometry to develop his ideas.
This apparently did not come easily to him, and involved a lot of consultation
with other people in Europe. He and Levi-Civita were in very frequent
communication for example. So he may have been responding to someones comment of
the sort we all hear that they we’re having a hard time with math.
댓글 없음:
댓글 쓰기